Since Hoppy decided to repost this picture from .org depicting what he calls a 12.9 ft drop, I'm going to repost my response here:
Here we go: Using the calculator on
this page we can determine how far the horizon is for a given elevation. So for 2 ft the distance to the horizon is 1.73 miles.
Which means you subtract the distance 1.73 miles from your 4.4 miles to get 2.67 miles.
Using rowbothams method for deriving the drop:
To find the curvature in any number of miles not given in the table, simply square the number, multiply that by 8, and divide by 12. The quotient is the curvation required.
2.67^2 * 8 / 12
This makes the new drop 4.75 feet.
And without a good image to indicate what that beach really looks like it is difficult to determine what is going on but I would guess that a typical beach like the one we see in the picture is at least over 5 ft.
Later on in the thread Silhouette29 posted a great picture to help hoppy understand why you don't simply subtract the height of 2 ft from Rowbotham's 12.9 ft to get the drop:
Like I said earlier with an example using 6 feet for the observation height. At 4 miles, one doesn't simply subtract that height from the drop (10-6), as that would indicate a 4 foot drop over the distance of 1 mile. It needs to be recalculated using the distance advantage of added height from the starting point.
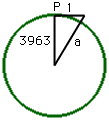
Using Rowbotham's diagram:

The drop is 4.75 feet and the image he posted sucks.
Also, note that in the picture he took there are some stark differences in topography when compared with the octagon house on Google Earth.
On Google Earth there is clearly a walkway and a lot of shrubbery in the way. This makes the picture highly suspect.