1

Flat Earth Theory / Re: Wiki - Tom Bishop Experiment

« on: June 06, 2019, 10:34:52 PM »

A YouTuber has spent, apparently, three years or so doing his own variations on the TBE, and today has (reluctantly, apparently) accepted that the Earth cannot be flat.

Didn't last long.

2

Flat Earth Theory / Re: Wiki - Tom Bishop Experiment

« on: May 28, 2019, 11:20:18 PM »

The claimed Bishop Observation was 23 miles from Monterey to Santa Cruz.

My observation in that graphic was 44 miles from La Jolla to San Clemente.

Different.

3

Flat Earth Theory / Re: Wiki - Tom Bishop Experiment

« on: May 28, 2019, 10:55:17 PM »

Another example of not seeing what should be seen if earth is flat:

In JTolan's now famous image of Mt San Jacinto taken from 120-ish miles away in Malibu, I can't see the Chino Hills at around 55-60 miles that should be visible if earth is flat. The elevation of the Del Rey bluffs just east of Santa Monica about 20 miles away from Malibu aren't tall enough to obscure the Chino Hills 55-60 miles away if earth's surface is a plane. Can't call it "dirty air" or "convergence zone" or "compression."

The foothills and shoulder of San Jacinto mountain are missing too. It's summit appears at a declined angle from where it should be if earth is flat.

You can do the "we can sometimes see farther than we should if earth is a globe" but I can more often say we can't see what we should if earth is flat.

4

Flat Earth Theory / Re: Wiki - Tom Bishop Experiment

« on: May 28, 2019, 10:40:05 PM »

I've yet to see "as far as we should" if the earth was flat.

Yes you did.

You then proceed to show me an example of when I DIDN'T see as far as I should if the earth was flat.

Do you see the beach in San Onofre in that imagery? I dont. If the earth was flat, I should  be able to see the coastline, and not just a hill higher up and farther away.

(That sighting, btw, maps to a globe earth with atmosphere. I don't live on a geometric spheroid with no air density gradient. But that's not the point. Maybe I do. Maybe the globe has a radius of 10,000 miles and that hill SHOULD be visible geometrically. The point is it isnt flat because I'm not seeing all that I should if the earth is truly flat. This is your argument turned around. Do you see that? Showing that something can be seen that supposedly shouldn't be seen if a globe doesn't logically mean the earth is flat. That sighting suggests convexity, not flatness.)

5

Flat Earth Theory / Re: Wiki - Tom Bishop Experiment

« on: May 28, 2019, 10:03:07 PM »

I've yet to see "as far as we should" if the earth was flat.

I can't reproduce what is claimed in the Bishop Antecdote even at half the distance. And yet you, Tom, say you did it often; anytime you had your doubts.

Every level alignment observation I've made suggests a convex surface. As impressive as a 17-mile mirror flash was, it's still not proving flat. I've done a 800' to 400' mirror test through thick haze over a 20 mile span with a small 3"×5" mirror. But the surface level record, on a clear day, with a 12"x48" mirror is only 17.5 miles. Is that "farther than it should" on a 3959 mile radius globe with an atmosphere? Maybe. But there are ways it could happen. Or maybe the globe is larger and the convexity less.

Do the mirror test across Monterey Bay from Santa Cruz. If you can play the "shouldn't be able to see" game then I'll play the "should be able to see" counter game. If there are atmospheric reasons why you can't see forever on a flat earth, there are atmospheric reasons why we can, sometimes, see farther than on a globe with no atmosphere.

6

Flat Earth Theory / Re: Wiki - Tom Bishop Experiment

« on: May 28, 2019, 08:25:14 PM »

What brand of coffee maker did you have 12 years ago?

If I'd have used it in citing "evidence" for some extraordinary claim, I'd sure as heck remember.

You mean except for the cars which were commented on as moving in the background?

Those vehicles weren't on the beach. The highway is higher.

Did you see Santa Cruz at all?

No.

7

Flat Earth Theory / Re: Wiki - Tom Bishop Experiment

« on: May 28, 2019, 06:20:43 PM »

You are talking about something that occurred 12 years ago. I no longer live in that area, nor do I have the telescope. It was a refracting Celestron that was advertising itself as 500x equivalent...

A Celestron model? In an old thread from a time closer to the original testimony you told folks asking this same question about what equipment you used that it was an Orion StarBlast 4.5".

Not that it makes a difference. It's just testimonial.  Not "experimental evidence."

Now, the QE2019 and Flat Reality Earth Explorers documented a successful signal mirror sun reflection from about 5 ft above the Salton Sea across a distance of 17.5 miles. Documented with video from an IR-modified P900. Pretty impressive. But other than the glint of mirror flash, absolutely no detail of the far shoreline. 

I was passing by Monterey a few weeks ago, hoping to attempt the "Bishop Experiment" with my Meade Infinity 90 refractory (2.6mm eyepiece), but too hazy. I've given up on it, and I find the continued inclusion of it in the Wiki to be simply an homage to the Society's most intrepid globe critic.

(Yes, I still lurk sometimes.)

8

Flat Earth Investigations / Re: Apparent size of the sun

« on: January 12, 2019, 10:06:14 PM »

My first contribution to the forum, almost 9 months ago.

https://forum.tfes.org/index.php?topic=9487.0

9

Flat Earth Investigations / Neumayer Station III

« on: January 12, 2019, 05:40:06 AM »

There's a 24-hour webcam posting previous 24 hour shots at 10-minute intervals, located on the Ekström ice shelf, drifting but currently at  S70°38′42″, W8°15′51″.

https://www.awi.de/en/expedition/stations/neumayer-station-iii.html

According to Stellarium, that location will not experience a sunset until January 25th. The camera is static so you can't watch the sun move around 360 degrees, but you could check it daily to see if it enters twilight. 


January 12th

10

Flat Earth Theory / Re: The Sagitta (Globe Earth "Theory")

« on: January 11, 2019, 04:15:24 AM »

Right.

The "bulge" is a useless measure, IMO. Worse, it gets misapplied by those on both sides.

I think I'll make this pitch to Mick West (Metabunk) and Walter Bislin. See what they say since their curvature calculators are so often used.

11

Flat Earth Theory / Re: The Sagitta (Globe Earth "Theory")

« on: January 10, 2019, 09:55:48 PM »

...redoing on my graphing calculator, I got a difference of 0.00007178 miles.

That's 4.5 inches.

Can that be right?  30 miles, and the difference between the distance over a globe earth with a bulge and a flat earth is only 4-5 inches?

12

Flat Earth Investigations / Re: Apparent size of the sun

« on: January 10, 2019, 09:53:13 PM »

I've tested it. I wore polarized sunglasses and looked at a car. The reflection of the sun did not disappear.

I wouldn't expect it to, with polarized sunglasses.

I'm not sure why you are looking for something to disappear or not disappear, but the difference with polarized filtering and full-spectrum light filtering means that you shouldn't expect one to perform the same as the other.

I guarantee you that if you see a reflection of sunlight on a car's surface, using a solar filter will make it completely disappear, along the car and everything else. It will be completely dark.

If you see a reflection of the sun in the windshield, unlike a polarized sunglass lens, a solar filter will knock out everything EXCEPT a small circle of the sun...the actual sun; not the glare, flare and blooming of light that appears around an unfiltered sun. Your polarized filter won't do that. It's not supposed to, which is why you are confused in equating polarization with light filtering.

In a little while, I'll go out and take photos of filtered and unfiltered reflected sun and show you what I mean.

Per your second question, Rowbotham provides an image in Earth Not a Globe.



In the above image the sun is projected on 6, 7, 8, 9 and 10.

One can also imagine the screen of the atmosphere as a "dome" around the observer rather than a plane.

Like the Bat Signal projecting onto clouds or haze in the sky?
Or like a movie being projected onto a screen from behind the screen?

The car reflection model/illustration you proposed is like the former.
ENaG suggests to me the latter.

Which is it?

13

Flat Earth Theory / Re: The Sagitta (Globe Earth "Theory")

« on: January 10, 2019, 09:09:20 PM »

There has to be some difference. The arc and chord length can't be equal. The arc length must be greater.

If using that calculator and the two values are the same, try changing to smaller units and/or adjusting the precision. If working with miles and the numbers coming out the same, that might suggest the answer is less than half a mile.

14

Flat Earth Theory / Re: The Sagitta (Globe Earth "Theory")

« on: January 10, 2019, 08:52:03 PM »

Thanks for giving this a whirl. So as not to confuse the issue, don't rely on any of my numbers to work it out. Just use 30 miles on the arc of a circle with radius of 3959 miles.

What's the difference between arc length and chord length? 1.7 miles?

15

Flat Earth Theory / The Sagitta (Globe Earth "Theory")

« on: January 10, 2019, 06:41:09 PM »

Sagitta is the fancy term for what is commonly referred to in flat vs. globe discussions as "the bulge."



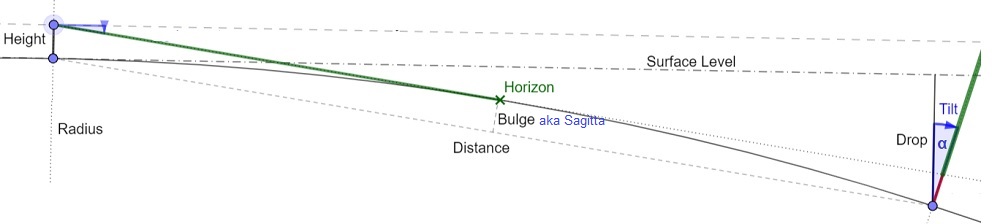
I've always been a bit annoyed by references to "the bulge" in talking about "globe earth theory;" and not because of the term itself but because I don't think the measure of the arc above the chord is germane. The bulge is not a mound or hill that rises up in front of you on a globe and presenting an obstacle. The bulge is not the obstacle. The horizon is.

The only time that the bulge matters is when the horizon happens to coincide with it, as I've depicted with the diagram (from Metabunk's interactive visualizer) above. The horizon is a function of height above the arc. The bulge is a function of base locations on the arc. For any two points on the arc of a globe, the obstructing horizon varies with height but the bulge is fixed.  Only at a height where the horizon corresponds to the midpoint on the arc between two points does the bulge matter, and then only because it's where the horizon is.

The "bulge" isn't a mound rising before your eyes. On a globe, the arcing surface drops away from your eye level. If I had my druthers, we'd banish "the bulge" from any discussion about how things work on a globe. It's often misconstrued and misrepresented as that which causes or would cause an obstruction to line of sight compared to a flat earth; but that's in error.

Consider two points on earth that are 30 miles apart.
On a flat surface, they're just that: 30 miles apart.
On a globe with a radius of 3959 miles, they are 30 miles apart on an arc of 0.43°. The height of that arc above a straight line (chord) between those two points -- that height being the bulge or sagitta -- is 0.0284 miles or 150 feet.  Over the span of 158,400' between the two points on the arc, how significant is that 150' sagitta?

That additional 150' of the sagitta (bulge) contributes some amount of additional distance on the arc of a globe compared to the straight line chord length which would be the flat earth distance. Guess how much extra distance? Or, if you know how to do the math, work it out.

I'll be back.

Edit: Come on, my fellow globelings. This question is for anyone, and I'm sure globe supporters can come up with the solution. There's probably even an online calculator that can provide the number.

But until then, just guess. Anyone? What do you think the difference is between arc length and chord length in the 30-mile distance scenario above that a 150' "bulge" would produce? Just ballpark it.


 

16

Flat Earth Investigations / Re: Apparent size of the sun

« on: January 10, 2019, 03:25:49 PM »

The sun is reflecting off of the side of a car. Will either a solar filter or a polarized lens, or any filter you choose, make the sun disappear off of the side of that car? If not, then it will do nothing for a reflection or projection and the matter is moot.

Test it.

Or do you want someone else to do it for you?

I'll do it. "Make the sun disappear." What does that mean? Glare of the reflection disappear or the entire reflected light from the sun?

If we have sun today, I'll take photos of the sun reflecting off a car (roof and windshield) through both polarized lens and solar filter. We'll see if either or both does nothing. If either does something, the matter won't be moot.

And while we're on the topic of modeling this "projected sun" notion, does this proposal suggest the sun and observer are on the same side of the projection plane? Or is the sun projection on the other side, like in seeing a movie from the opposite side of the screen. I'm still trying to work out the orienting of the projection on the atmoplane concept.

17

Flat Earth Investigations / Re: Apparent size of the sun

« on: January 10, 2019, 03:04:56 AM »

A solar filter only reduces the glare in your eyes, for eye protection, it wouldn't eliminate an external projection on the atmosphere.

Again with the unexplained assertion about "projection on an atmosphere." Are we ever going to get an explanation of how that phenomenon works or how it can make the sun appear laterally displaced from where it is "projecting" from?

Does wearing sunglasses in a movie theatre shrink or eliminate the projection of the movie on the screen?

Is the movie on the screen so bright that you can't see what's on the screen and the whole front wall is lit up too bright to look at?

When I apply my solar filter to the lens of my camera, the sun doesn't keep shrinking the more I limit exposure. The outline of the orb "shrinks" to its actual size and that's the end of the "shrinking." I can get it dimmer and redder, but with the filter applied, I now see the actual sun and not the burst of white light that exceeds the dynamic range of my sensor and hus occupying an inordinately large area in the field of view.

Simply applying polarized filtering doesn't do the trick. Headlamps and polarized glasses are not the analogy for knocking down the gain of the sun so that you can view its actual shape and size. Properly filteredt, you can track the sun (or its "projection" if you insist) and it doesn't magnify or shrink as it moves toward setting.

That can be shown zetetically. It's contrary to zeteticism to draw conclusions based on ad hoc speculation, and use flawed analogies or illustrations to bolster such speculation.

To remind you of your previous insistence on the burden of proof for a positive claim, the sun being a projection on the atmoplane is false until you prove it.

You have asserted a claim without proof. You've tried to explain it  via a bad analogy. But despite my challenge to you to explain it, how the mechanism works, and how it can explain phenomenon we can observe, you persist on dodging in favor of simply repeating the ad hoc assertion in a new thread.

If it were me doing that, you know what would happen. All I can do is pester you.

18

Flat Earth Investigations / Re: Apparent size of the sun

« on: January 09, 2019, 10:23:53 PM »

Prove that the flare is in the camera lens and that it's not an enlargement in the sky. The article also includes versions of the effect as seen in polarized lenses.

It appears that the article has provided evidence, while you have provided none. A massive failure to persuade or to support your argument on your part.
[/quote]

Polarized lenses on headlights?

Try a solar filter on the actual sun:



Actual sun not changing size, seen through a solar filter:


There's some proof. And it's not a flawed analogy of headlights and polarized glasses.

19

Flat Earth Investigations / Re: More on "13 Miles: 60 ft NOT Hidden".

« on: January 08, 2019, 04:15:55 PM »

I haven't cracked the code on when to expect inferior mirage or not. Some days it's absent. More often than not, it's there though. Sometimes strong like on this day. Other days weak. But looking at air temp, sea temp, time of day doesn't afford me anything predictive.

What I want to know is what is behind the inverted image of the mirage? If you could erase it, would you see more of the distant land below that line where the mirror flash became obscured? Or you see the sea rise and reveal itself to be what is obscuring the light? I think I understand the answer, but I don't know for sure. I'll just have to keep doing this, hoping for another clear day when there is no distortion by mirage.

Not what I expected.





Red-outlined arrow is where the lowest point the mirror could be seen during mirage conditions. The (near) absence of inferior mirage reveals about 5-7' more of the stairs and maybe the tip of the guard tower roof.  This is a screen capture from a video I shot yesterday evening about 30-45 minutes before sunset. Tide was just turning on a -0.6 low.

20

Flat Earth Theory / Re: Solar spotlight

« on: January 07, 2019, 10:35:40 PM »

I'll hazard an attempt to articulate what Tom has been attempting to say is the explanation.

It's not a "literal spotlight."  It's not the sun that is shaded from illuminating in certain directions. It's the atmo- (air) through which the sun is shining that is doing the shading.

When the sunlight has to penetrate less air mass, that is when we see it "projected upon the atmoplane." But at longer distances, when the angle of the sun is shallower, the air eventually becomes opaque to the sun and hence the sunlight won't penetrate it and for those in darkness, there is no "projection upon the atmoplane" due to that opaqueness.

This is not the cause of sunset. That's either a perspective effect or light bending upward. Even after sunset (or before sunrise) there is twilight because the sun's light is beginning to penetrate the air as the angle becomes shallower and thus less air to pass through.

If I have that wrong, I apologize; I can't claim to be able to fully apprehend it since I give it so little credence. It's more like a meteorological explanation than an atmoplanar one, and day/night would vary due to weather more so than characteristic atmo- opacity.

There's also the issue of the magnification effect claimed for which the atmolayer, with increasing density, magnifies the sun such that it maintains its apparent angular size despite receding into the distance. It's a remarkable confluence of features: atmolayer shading, magnification and perspective (or EAT) that causes the sun to appear to rise and set while all the while actually being some distance over a flat earth.

And this still doesn't explain the lateral displacement of the projected sun from the actual sun, but that's an issue pending explanation on another topic. Still, I hope I've gotten the "spotlight" "projection on the atmoplane" thing right.